Richard M. Christensen |
||
I. PURPOSE AND CONDITIONS
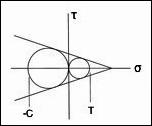
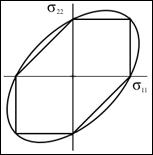
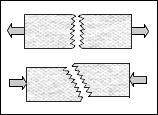
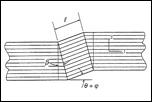
Well constructed failure theories can discriminate safe states of stress in materials from states of certain failure, based upon calibration by a minimal number of failure type mechanical properties. The specific purpose here is to provide failure criteria for general types of materials Two of the conditions that are taken to apply are those of a macroscopic scale of consideration as well as the corresponding macroscopic homogeneity of the material. The concepts of macroscopic scale and macroscopic homogeneity have connotations familiar to everyone. However, trying to define these concepts in absolute terms is extremely difficult. Macroscopic homogeneity is taken to be the condition that the materials constitution is the same at all locations. Thus the problem is shifted to the precise meaning of the term location, which depends upon the scale of observation. Suffice to say, the scale of observation is taken such that all the common forms of materials are included, such as metals, polymers, ceramics, glasses and some geological materials. Materials which are excluded are porous materials, whether cellular or not, as well as granular materials. In the case of metals, the macroscopic scale must be taken as being much larger than the size of the individual grains, presumably about an order of magnitude larger. Thus the macroscopic scale depends upon the type of material. For polymers, it must be much larger than the characteristic molecular dimension. Other cases are similarly apparent. Effects at the nano-meter and micro-meter scales do not explicitly enter the macroscopic formulation. To attempt to bring in these effects would add parameters restricted to a particular materials type and obscure the clarity of a self contained and self consistent treatment at the macroscopic scale. However, after obtaining the general macroscopic treatment it is very interesting to compare and interpret with effects at the smaller scales for specific materials types. Failure itself can sometimes be difficult to identify and even more difficult to define. For cases considered here, failure is taken to involve the interruption of the usual linear, reversible range of behavior by a major change to irreversibility. Failure implies the materials lack of ability to sustain and support significant loads. In subsequent sections the term damage will be defined as a subset of failure. Failure in solids bears an interesting relationship to turbulence in fluids. Fully turbulent flow in fluids and the failure occurrence in solids marks the departure from linear control to a completely nonlinear behavior. It does not appear possible to establish an analogy between turbulent flow and failure in generalized continua, nevertheless both cases conform to the dominance by an ultimate nonlinearity. In effect both cases represent completion of the account of behavior prescribed by the balance laws and constitutive equations. Some perspective may be appropriate on the effort to theoretically characterize the failure of materials of various types through mathematical criteria. Perhaps no other scientific quest had as much energy expended upon it historically with so little to show for the effort. The viewpoint here holds that the scientific advances of the modern era lays out the proper tools and directions which can finally bring failure criteria to a level of unification (and turbulence modeling as well). The insight offered by fracture mechanics is especially helpful in understanding brittle failure in homogeneous materials. Several qualifications must be included concerning the purpose and scope of this website. It is not aimed as a literature survey, the goal here is to be technically discriminating rather than inclusive. The field of materials failure in general and failure criteria in particular is enormously broad. A complete treatment of such matters would be unrealistic and probably impossible. The focus here here is upon the theoretical basis for the specific failure criteria under consideration. By the term “theoretical basis” is meant the physical and mathematical underpinnings of the various failure forms. No attention will be given to empirical forms which may seem to work well in certain situations but have no foundation from which to judge their likely generality. Although it is not intended to integrate specific data sets for particular materials, which itself is a large and important but self contained topic, nevertheless guides and references to such sources will be given wherever possible. Finally, all failure criteria to be reviewed here are taken directly from (or intimately related to) results already presented in peer reviewed, archive journals. Such publications have received critical examination and evaluation. While this certainly does not provide any kind of warranty, it does help to assure fidelity with important aspects of physical behavior, which is the central objective here. With this emphasis on substance and reliability, the present web based account is intended as a resource for all those having commitment to materials science and materials applications. The next section will be on failure criteria for homogeneous and isotropic materials. Other sections will be added later starting with the case of highly anisotropic fiber composite materials. Comments are welcome on anything covered herein.
Richard M. Christensen |
|||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||
Recent Additons |
|||||||||||||||||||||||||||||||||
Key Junctures |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
General Matters |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
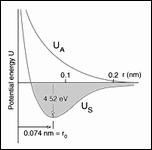
Can Atomic/Nano Scale |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
||||||
 |
||||||
 |
||||||||
 |
||||||||
 |
||||||
Copyright© 2019 |
||||||



