Richard M. Christensen |
||
Is it Stress or Strain That Causes Failure
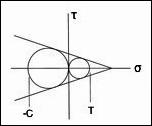
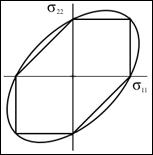
It is likely that the stress versus strain issue will remain as a strongly held division between the practitioners for some time to come. Experience often involves trade-offs, contradictions and anomalies. However, at the research base there should be a logical resolution of the problem. The most commonly used form is that of the Mises criterion expressed in terms of stress. Other criteria also in common usage are the maximum shear stress criterion (Tresca), the maximum normal stress criterion, the maximum normal strain criterion, and dilatational criteria in terms of either stress or strain. The Mises criterion applies to isotropic materials, and therein lies an unforeseen complication. The stress strain relations for isotropy can be used to convert the Mises criterion in terms of stresses into a criterion expressed in terms of strains. A surprising thing happens, the strain form is the same as the stress form. In other words the Mises criterion is form invariant between stresses and strains. It is a short step from the above incontrovertible fact to assume that all criteria can be switched from stress to strain (and visa versa) with no basic change in character. Such is not the case however, especially when considering the broader classes of anisotropic forms but also for some isotropic cases. As an example, the maximum normal stress criterion and the maximum normal strain criterion are of decidedly different forms when interconverted. Another fact to be considered is that the Mises criterion is only applicable to ductile metals and it is in serious error for all other materials types. These matters signal the difficulties that ensue in trying to go from stress to strain or the reverse in considering failure. All of these complications trace back to the tensor valued nature of stress and strain. If they were scalars the difference would be trivial. A rational basis is needed for making the judgment call on the stress versus strain uncertainty. In this website, and in the supporting peer reviewed papers upon which it is built, stress is taken as the fundamental form to be used with failure criteria. This is the direct consequence of the following conditions. Stress must be used if one wishes to have compatibility with fracture mechanics in the brittle range and with dislocation dynamics in the ductile range. Both of these classical theories require formulations in terms of stress. To not have union with these two anchor points of physical reality would impose major difficulties, practically as well as conceptually. In a totally different direction, a very interesting failure effect is exhibited by materials with time dependence (even an almost undetectable degree of time dependence as with polymers below the glass transition temperature). For the application of constant load (stress) the situation is termed as creep. If the load is maintained long enough, even years in some cases, at some point total failure can occur, creep rupture. Conversely, when a state of time constant strain is applied, termed as relaxation, no failure occurs no matter how long the strain state is maintained. Even the technical term “relaxation” is descriptive of what is happening at the molecular scale in the case of constant strain. Clearly, stress rather than strain is the stimulus for failure in these problems. Yet another indication of the more sound basis for stress is that it is applicable both to solid materials and to fluids. Strain (deformation) is not, it has no meaning for fluids. Some viscoelastic fluids can and do fail, stress necessarily must be used in such cases. Stress, not strain, will be used here in characterizing failure criteria for the physical reasons just stated. This decision will be further substantiated by the success of stress based forms and the ambiguity of strain based forms in particular examples. |
|||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||
Recent Additons |
|||||||||||||||||||||||||||||||||
Key Junctures |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
General Matters |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
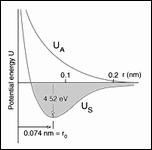
Can Atomic/Nano Scale |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
|||||||||||||||||||||||||||||||||
 |
||||||
 |
||||||
 |
||||||||
 |
||||||||
 |
||||||
Copyright© 2019 |
||||||



